In this lesson I’ll be looking to explain everything you’ll ever need to know about intervals in less than 15 minutes. We’ll look at the basic set of intervals derived from the major scale, minor intervals, augmented and diminished intervals, compound intervals and inverted intervals.

Interval Basics

First let’s lay out three basic points regarding intervals:

1. Intervals are measurements of musical distance like tones and semitones (whole steps and half steps). Intervals are simply a little more descriptive.

2. Interval names are unrelated to scales. The basic set of intervals are roughly named according to the major scale but, beyond that, ignore any relationship to scales.

3. Intervals are basically the alphabet of music. They are the letters of the language. Musical phrases, scales, chords, arpeggios and everything else can be described and built from intervals.

Intervals Of The Major Scale

The most common set of intervals are based on a major scale. Below we see a simple C Major Scale:

We can then number the notes of the scale:

Intervals also contain a quality. The intervals of the major scale contain the following qualities:

Simply follow the principles:

• 1^st, 4^th and 5^th are all Perfect Intervals
• All the rest are Major

Minor Intervals

To create a Minor Interval we simply take a Major Interval and drop the top note by a half step.

Here we see that from a root note of C, a Major 3^rd is E and a Minor 3^rd is Eb:

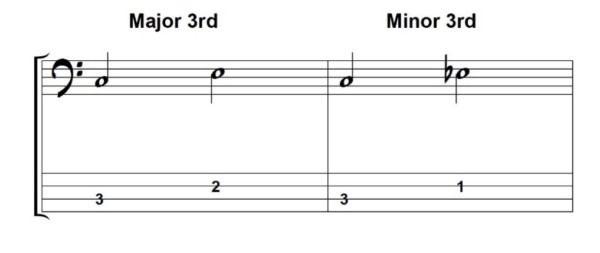
We can apply this principle to every Major interval and create Minor 2^nd, Minor 3^rd, Minor 6^th and Minor 7^th intervals:

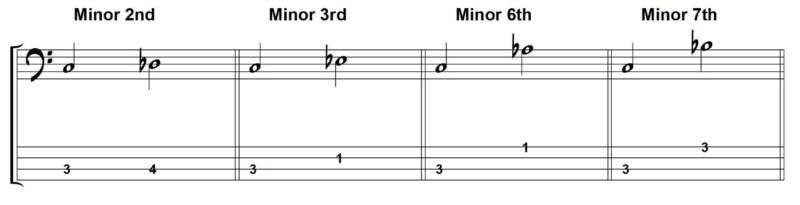
Minor Intervals tend to sound darker or ‘sadder’. By listening out for the Emotive Quality of each interval we can develop our musicianship, ear and musical vocabulary.

Augmented & Diminished Intervals

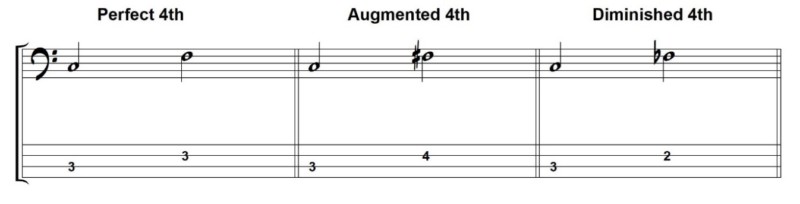
We generally create Augmented and Diminished intervals by raising or lowering the Perfect Intervals by a half step.

• An Augmented Interval is created by raising the top note of a Perfect interval by a half step
• A Diminished Interval is created by lowering the top note of a Perfect interval by a half step

Here we can see these intervals applied to the 5^th:

Here we can see these intervals applied to the 4^th:

Enharmonic Equivalency

With a lot of augmented and diminished intervals we can find ourselves using the same pitch but with a different note name.

For example, the Diminished 4^th from C is Fb. This could also be written as C to E.  However, if we look at the notes alphabetically:

• D is the 2^nd note from C
• E is the 3^rd note from C
• F is the 4^th note from C etc.

Therefore, C to E is always a 3^rd of some kind. C to F is always a 4^th of some kind, regardless of the sharps or flats involved.

For this reason, C to Fb is a Diminished 4^th and C to E is a Major 3^rd. They are the same pitch and fret on the bass but the interval name is different because of their alphabetic placement.

This principle of ‘same pitch – different note name’ is called Enharmonic Equivalency.

More Augmented/Diminished Intervals!

As well as augmented and diminished intervals on the 1^st, 4^th and 5^th we can also have augmented and diminished 2^nd, 3^rd, 6^th and 7^th intervals! These are much less common but still an important part of music theory.

The augmented and diminished intervals lie above the major and below the minor:

• An Augmented Interval is created by raising the top note of a Major interval by a half step
• A Diminished Interval is created by lowering the top note of a Minor interval by a half step

Below are some examples of these intervals:

On a root note of A we could have the following 6^th intervals:

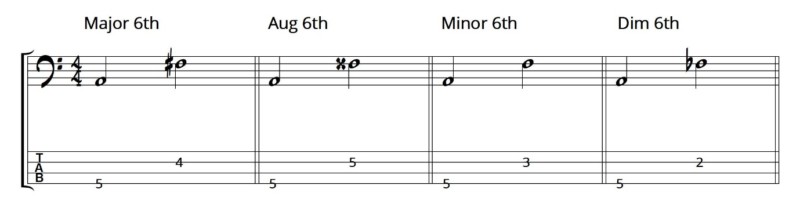
• A to F#: Major 6^th
• A to F##: Augmented 6^th
• A to F: Minor 6^th
• A to Fb: Diminished 6^th

On a root note of D we could have the following 7^th intervals:

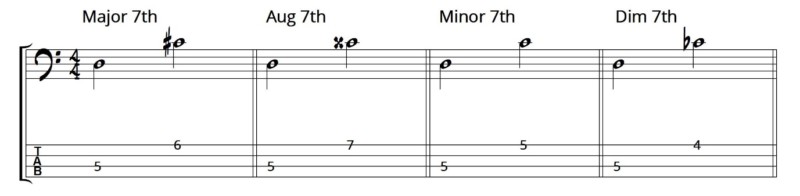
• D to C#: Major 7^th
• D to C##: Augmented 7^th
• D to C: Minor 7^th
• D to Cb: Diminished 7^th

Beyond The Octave: Compound Intervals

So far, we’ve only looked at intervals within an octave. But we can also move to infinity and beyond by looking at Compound Intervals.

These are intervals beyond the octave and are easy to understand because they’re exactly the same as the intervals we’ve already covered but they just have a higher number.

The Octave is an interval in its own right: the perfect 8^th.

Beyond the 8^th we can continue our set of intervals as the 9^th, 10^th, 11^th, 12^th, 13^th, 14^th and the 15^th as the second octave.

These intervals correspond to the intervals in our first octave:

• 9^th = 2^nd
• 10^th = 3^rd
• 11^th = 4^th
• 12th = 5^th
• 13^th = 6^th
• 14^th = 7^th
• 15^th = 8^th (octave)

By applying the interval quality, we can see the set of intervals within the major scale as:

• Perfect 8^th (Octave)
• Major 9^th
• Major 10^th
• Perfect 11^th
• Perfect 12^th
• Major 13^th
• Major 14^th
• Perfect 15^th

The compound intervals in the second octave can be seen below from a C note. Remember, there are many different fingerings for any one interval. These are just an example:

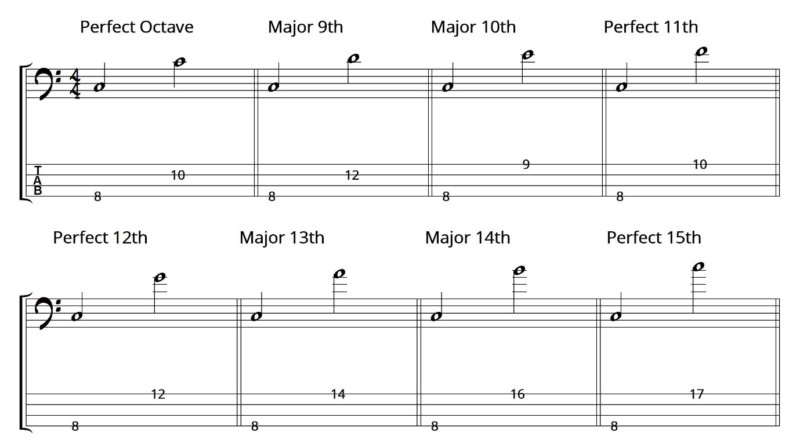
These intervals can also be labelled by simply inserting the prefix ‘compound’. For example, the Major 9^th may be written as Compound Major 2^nd or the Perfect 11^th would be the Compound Perfect 4^th.

Interval Inversion

Lastly, we need to look at interval inversion. When we invert intervals we are basically measuring them in a downward direction. As an example:

• C up to B is a Major 7^th
• C down to B is a Minor 2^nd

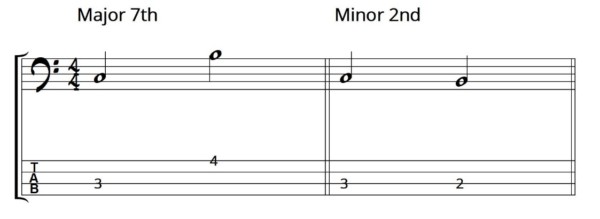
This is interval inversion. We measure the same two notes but in the opposite direction.

The rules for interval inversion are as follows:.

• 2^nd becomes 7th 
• 3^rd becomes 6th 
• 4^th becomes 5th

And vice versa:

• 7^th becomes 2^nd
• 6^th becomes 3^rd
• 5^th becomes 4^th

For the qualities:

• Perfect Intervals stay the same
• Major Intervals become Minor (and vice versa)
• Augmented Intervals become Diminished (and vice versa)

Here are some examples:

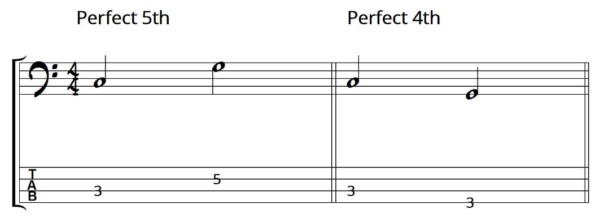
• C to G ascending is a Perfect 5^th
• C to G descending is a Perfect 4^th

• D to F ascending is a Minor 3^rd
• D to F descending is a Major 6^th

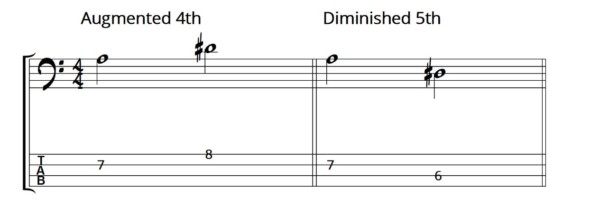
• A to D# ascending is an Augmented 4^th
• A to D# descending is a Diminished 5^th